How To Draw A Phase Plane

Prove Mobile Notice Bear witness All NotesHide All Notes

Mobile Notice

You appear to be on a device with a "narrow" screen width (i.east. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best views in landscape mode. If your device is not in mural mode many of the equations will run off the side of your device (should exist able to gyre to see them) and some of the bill of fare items will be cutting off due to the narrow screen width.

Section 5-6 : Phase Plane

Earlier proceeding with really solving systems of differential equations there's one topic that we demand to take a await at. This is a topic that's not always taught in a differential equations class but in case you lot're in a course where information technology is taught we should comprehend it so that yous are prepared for information technology.

Let's start with a full general homogeneous system,

\[\begin{equation}\vec x' = A\vec x\characterization{eq:eq1}\end{equation}\]

Discover that

\[\vec x = \vec 0\]

is a solution to the arrangement of differential equations. What we'd like to ask is, exercise the other solutions to the arrangement approach this solution every bit $t$ increases or exercise they move abroad from this solution? We did something similar to this when we classified equilibrium solutions in a previous department. In fact, what we're doing here is merely an extension of this idea to systems of differential equations.

The solution $\vec x = \vec 0$ is chosen an equilibrium solution for the arrangement. Equally with the single differential equations example, equilibrium solutions are those solutions for which

\[A\vec x = \vec 0\]

We are going to assume that $A$ is a nonsingular matrix and hence will have just 1 solution,

\[\vec x = \vec 0\]

and so nosotros volition have simply ane equilibrium solution.

Back in the single differential equation instance recall that we started by choosing values of $y$ and plugging these into the function $f(y)$ to decide values of $y'$. We and then used these values to sketch tangents to the solution at that particular value of $y$. From this we could sketch in some solutions and use this information to allocate the equilibrium solutions.

Nosotros are going to do something similar here, but it volition be slightly different besides. First, nosotros are going to restrict ourselves down to the $two \times ii$ case. So, we'll exist looking at systems of the form,

\[\begin{array}{*{20}{c}}\brainstorm{align*}{{x'}_1} & = a{x_1} + b{x_2}\\ {{x'}_2} & = c{x_1} + d{x_2}\terminate{align*}&{\hspace{0.25in} \Rightarrow \hspace{0.25in}\hspace{0.25in}\vec x' = \left( {\begin{array}{*{20}{c}}a&b\\c&d\end{array}} \right)\vec x}\end{assortment}\]

Solutions to this system will be of the grade,

\[\vec 10 = \left( {\begin{array}{*{20}{c}}{{x_1}\left( t \right)}\\{{x_2}\left( t \right)}\end{assortment}} \right)\]

and our single equilibrium solution volition be,

\[\vec ten = \left( {\brainstorm{array}{*{20}{c}}0\\0\end{array}} \correct)\]

In the single differential equation case we were able to sketch the solution, $y(t)$ in the y-t plane and encounter actual solutions. Even so, this would somewhat difficult in this instance since our solutions are actually vectors. What we're going to do here is think of the solutions to the system every bit points in the ${x_1}\,{x_2}$ airplane and plot these points. Our equilibrium solution will stand for to the origin of ${x_1}\,{x_2}$. aeroplane and the ${x_1}\,{x_2}$ plane is called the phase plane.

To sketch a solution in the phase plane we tin pick values of $t$ and plug these into the solution. This gives us a point in the ${x_1}\,{x_2}$ or phase plane that we tin can plot. Doing this for many values of $t$ will and so give us a sketch of what the solution volition be doing in the phase plane. A sketch of a item solution in the phase plane is called the trajectory of the solution. Once nosotros have the trajectory of a solution sketched we can and then inquire whether or not the solution will approach the equilibrium solution as $t$ increases.

We would like to be able to sketch trajectories without actually having solutions in hand. At that place are a couple of ways to do this. Nosotros'll look at one of those here and nosotros'll look at the other in the side by side couple of sections.

One way to go a sketch of trajectories is to do something similar to what we did the first time we looked at equilibrium solutions. We can choose values of $\vec x$ (notation that these volition be points in the stage plane) and compute $A\vec x$. This will give a vector that represents $\vec ten'$at that item solution. As with the single differential equation case this vector will be tangent to the trajectory at that betoken. We can sketch a bunch of the tangent vectors and then sketch in the trajectories.

This is a fairly work intensive mode of doing these and isn't the way to do them in general. However, it is a mode to get trajectories without doing any solution piece of work. All we demand is the organization of differential equations. Let's take a quick look at an example.

Example i Sketch some trajectories for the system, \[\begin{array}{*{twenty}{c}}\begin{align*}{{x'}_1} & = {x_1} + 2{x_2}\\ {{ten'}_2} & = iii{x_1} + two{x_2}\end{align*}&{\hspace{0.25in} \Rightarrow \hspace{0.25in}\vec x' = \left( {\begin{assortment}{*{20}{c}}1&ii\\3&ii\end{array}} \right)\vec x}\end{array}\]

Show Solution

So, what nosotros need to do is selection some points in the phase aeroplane, plug them into the correct side of the organization. We'll practice this for a couple of points.

\[\begin{align*}\vec 10 & = \left( {\begin{assortment}{*{xx}{c}}{ - 1}\\ane\end{array}} \right) & \Rightarrow \hspace{0.25in}\vec x'& = \left( {\begin{array}{*{20}{c}}1&2\\3&ii\end{assortment}} \right)\left( {\begin{array}{*{20}{c}}{ - 1}\\1\finish{array}} \right) = \left( {\begin{assortment}{*{twenty}{c}}1\\{ - ane}\end{array}} \right)\\ \vec ten & = \left( {\begin{assortment}{*{20}{c}}2\\0\end{array}} \right) & \Rightarrow \hspace{0.25in}\vec 10' & = \left( {\begin{array}{*{20}{c}}i&2\\3&ii\finish{assortment}} \right)\left( {\brainstorm{array}{*{20}{c}}2\\0\end{assortment}} \right) = \left( {\begin{assortment}{*{twenty}{c}}2\\6\end{array}} \right)\hspace{0.25in}\\ \vec 10 & = \left( {\begin{array}{*{20}{c}}{ - 3}\\{ - two}\end{array}} \right) & \Rightarrow \hspace{0.25in}\vec ten' & = \left( {\begin{assortment}{*{xx}{c}}i&2\\3&2\end{array}} \right)\left( {\begin{assortment}{*{20}{c}}{ - iii}\\{ - 2}\end{array}} \right) = \left( {\begin{array}{*{20}{c}}{ - 7}\\{ - xiii}\stop{array}} \right)\hspace{0.25in}\stop{align*}\]

So, what does this tell us? Well at the point $\left( { - one,one} \right)$ in the phase aeroplane there will be a vector pointing in the management $\left\langle {ane, - 1} \correct\rangle $. At the point $\left( {2,0} \right)$ in that location volition be a vector pointing in the direction $\left\langle {2,6} \right\rangle $. At the point $\left( { - three, - 2} \right)$ there will exist a vector pointing in the management $\left\langle { - 7, - 13} \right\rangle $.

Doing this for a large number of points in the phase airplane will give the following sketch of vectors.

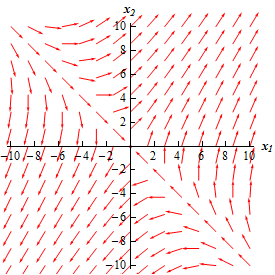
$A graph with domain $-10 \le x_{1} \le 10$ and range $-10 \le x_{2} \le 10$. This graph has a vast number of arrows on it. In the 1st quadrant the arrows all point generally towards the upper right corner and make approximately a 45 degree angle with the horizon. In the 3rd quadrant all the arrows all point generally towards the lower left corner and make approximately a 45 degree angle with the horizon. In the 2nd quadrant along an imaginary line y=-x (not shown) are a series of arrows that would fall on this line all pointing directly at the origin. Arrows that start just above this line all generally follow the same directly but are not pointed slightly to the right of the origin. As we move away from the line (still above it) the arrows start to point more directly into the 1st quadrant and by time we reach the $x_{2}$-axis the arrows are starting to look like the arrows that are in the 1st quadrant. Arrows that start just below the imaginary line all generally follow the same directly but are not pointed slightly to the left of the origin. As we move away from the line (still below it) the arrows start to point more directly into the 3rd quadrant and by time we reach the $x_{1}$-axis the arrows are starting to look like the arrows that are in the 3rd quadrant. In the 4th quadrant the arrows are similar to those in the 2nd quadrant. Along an imaginary line (not shown) y = -x arrows point directly towards the origin. Arrows that start just above this line all generally follow the same directly but are not pointed slightly to the right of the origin. As we move away from the line (still above it) the arrows start to point more directly into the 1st quadrant and by time we reach the $x_{1}$-axis the arrows are starting to look like the arrows that are in the 1st quadrant. Arrows that start just below the imaginary line all generally follow the same directly but are not pointed slightly to the left of the origin. As we move away from the line (still below it) the arrows start to point more directly into the 3rd quadrant and by time we reach the $x_{2}$-axis the arrows are starting to look like the arrows that are in the 3rd quadrant.$

Now all we need to do is sketch in some trajectories. To practise this all we need to exercise is remember that the vectors in the sketch above are tangent to the trajectories. Also, the direction of the vectors give the management of the trajectory every bit $t$ increases and so we can testify the time dependence of the solution past adding in arrows to the trajectories.

Doing this gives the following sketch.

$A graph with domain $-10 \le x_{1} \le 10$ and range $-10 \le x_{2} \le 10$. This graph has a vast number of arrows on it. This graph has exactly the same set of arrows as the previous graph. To avoid making this alt text too long we will not describe those again here. Also on the graph are a series of trajectories. First, there are two lines given by approximately y=-x and y=3/2x. Along the y=-x line are arrow heads that point towards the origin. Along the y=3/2x line are arrow heads pointing away from the origin. These two lines form a giant$

This sketch is called the phase portrait. Usually phase portraits only include the trajectories of the solutions and not whatsoever vectors. All of our phase portraits class this point on will merely include the trajectories.

In this case it looks like nigh of the solutions will outset away from the equilibrium solution then as $t$ starts to increase they move in towards the equilibrium solution and then eventually kickoff moving away from the equilibrium solution again.

At that place seem to be 4 solutions that have slightly dissimilar behaviors. Information technology looks like 2 of the solutions will start at (or near at least) the equilibrium solution and and so motion straight away from it while two other solutions start abroad from the equilibrium solution and then move straight in towards the equilibrium solution.

In these kinds of cases we call the equilibrium indicate a saddle betoken and we call the equilibrium signal in this case unstable since all but 2 of the solutions are moving away from it as $t$ increases.

As we noted earlier this is not generally the style that nosotros will sketch trajectories. All nosotros really need to go the trajectories are the eigenvalues and eigenvectors of the matrix $A$. We volition see how to do this over the adjacent couple of sections as we solve the systems.

Here are a few more than phase portraits so you can see some more possible examples. Nosotros'll actually be generating several of these throughout the course of the next couple of sections.

$This graph has no domain or range specified. The horizontal axis is labeled $x_{1}$ and the vertical axis is labeled $x_{2}$. The graph is labeled$ $This graph has no domain or range specified. The horizontal axis is labeled $x_{1}$ and the vertical axis is labeled $x_{2}$. The graph is labeled$

Not all possible phase portraits have been shown hither. These are here to show you some of the possibilities. Make sure to notice that several kinds can be either asymptotically stable or unstable depending upon the direction of the arrows.

Notice the difference between stable and asymptotically stable. In an asymptotically stable node or screw all the trajectories will motion in towards the equilibrium point equally t increases, whereas a heart (which is always stable) trajectory will just motility effectually the equilibrium point but never really motion in towards it.